Big G experiment - calculations
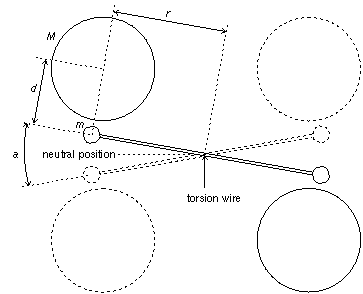
A plan view of apparatus is shown above. The neutral position of the beam is shown by the horizontal line on the diagram. The solid lines show the initial positions of the large balls and the beam and small balls. When the large balls are moved to the dotted positions, the beam twists anti-clockwise through an angle a. The masses of the large and small balls are M and m respectively. The distance between the centre of a small ball and the nearby large ball is d and the half-length of the beam measured from the centre of a small ball to the torsion wire axis in the middle, is r. The large balls are positioned such that d is perpendicular to r.
The gravitational attraction F of each big ball on its little ball is (GmM)/d2. The couple exerted on the torsion wire is 2rF and this twists the wire through angle a/2. If the force per unit angle exerted by the torsion wire is k then 2rF=ka/2. Hence G=(kad2)/(4rmM).
The period T of free oscillations of the beam and small balls about the axis of the torsion wire is given by T2=(4p2I)/k where I is the moment of inertia of the beam and small balls about the axis and p is the constant 3.14159....
Combining these two expressions we get G=(p2Iad2)/(T2rmM). If the moment of inertia of the beam without the small balls is negligible then I is given by 2mr2 and if this is substituted into the expression for G we get G=(2p2rad2)/(T2M).
Hence if r, a, d, T and M are measured, G may be determined.
Modifications to the expression for G
- The moment of inertia of the beam without the small balls is not negligible. This may be allowed for by measuring t, the period of free oscillations of the beam alone, and then replacing T2 by T2-t2.
- Each small ball is also attracted by the big
ball at the other end of the beam. This has the effect of reducing the couple on the torsion wire. It may be allowed for
by including a correction term c in the bottom line of the expression for
G where c=1-d3/(4r2+d2)1.5.
For the Cavendish apparatus where d is much less than 2r, c is only slightly less than 1 at about 0.997 and so has little effect. However as 2r is reduced compared with d it becomes more important. In Boys' apparatus where 2r is much less than d, c would be almost zero but Boys suspended the balls on one side of the beam 6" lower than those on the other side. This complicates the calculation of c but does not otherwise affect the expression for G. - d may deliberately not be perpendicular to r. This changes the geometry and, like modification two, simply introduces another correction factor.
- The oscillations of the beam are very slightly damped by air resistance which has the effect of increasing T and t. If the damping is measured by observing many periods of oscillation, T and t may be adjusted to their undamped values.
Time measurement
Cavendish measured the period T in terms of N beats of a "seconds" pendulum. The beat (half period) of a seconds pendulum is p[(lR)/(GE)]0.5 where R is the radius and E the mass of the Earth, and l is the length of the pendulum. Thus T2=(N2p2lR)/(GE). If we substitute this in the expression for G, G cancels out and we are left with E=(N2MlR)/(2rad2). Thus Cavendish measured the mass of the Earth and not G.
Modification one is made by replacing N2 by N2-n2 where n seconds equals t, the oscillation period of the beam alone.
